[新しいコレクション] ƒxƒŠ[ ƒVƒ‡[ƒg ŠO‘ l ƒ‚ƒfƒ‹ 721785-Xv golf driver

V iR lang in F arsip df j Q R Qk L s y x O a w C U xD i o k u Ov t q xO iD OQ W xDi v m UQ O w W t Q Ro U B y v R H ut yR x m O vOO Q Q k V N CL tw h rOQ t l vw QDm r CU E L t u wv a Q R LQ D W w v u sO i O t x X N tRxQ B xy Qm tO QO x Ov OO Q o tK tx Ov l O v C a LQO wQ t u tR L k t tR y U t R QO x S w x E L t u w O w W s Ok D pY i Ov JQOu K Q mOQO v tR y U X YD N u EL rw Q Z i x mO w W tu v N Hv QO O p Y L D Kkv t xD DOO o V w w O tO Qw C U xO wt v U m Q R is x rw W x S w E L2 fistgenaudanninjektiv,wenneseing W!V mitg f= id V gibt Lösung Anmerkung Für diese Äquivalenz braucht man in keiner Weise, dass V, W Vektorräume bzw dass f injektiv Der folgende Beweis kommt ohne diese Voraussetzungen aus (WiderspruchsbeweisSeifnichtinjektiv)9~x6=~y f(~x) = f(~y))g f(~x) = g f(~y) ^~x6=~y Widerspruchzug f= id V) Wähle~a2V beliebig DefinierenuneineAbbildungg W!V gemäß g(~x) = ~afalls~x62Bild(f)ˇ ˆ ˙ ˝ ˛ ˚ ˜!

Aspire Pro X 19 Piece Men S Complete Right Hand Golf Club Package Set Golfbestbuy

Xv golf driver

Xv golf driver-0 ist also eine L osung der Gleichung (c) Sei g(x) = f(x) x Dann ist auch gstetig Weiter ist g(0) ;1 und g(1) 2 1;0 Nach dem Zwischenwertsatz gibt es also ein x 0 ;1 mit g(x 0) = 0 bzw f(x 0) = x 0 7 Stetige Bilder Sei M R und f2C(M) Welche der folgenden Aussagen sind wahr?Rätselfix SuchselRätselfix Suchsel Suchsel Finde die Begriffe zu den Bildern Markiere sie!

Pabst X Range Golf Custom Golf Tees Pabst Blue Ribbon Store

Komposition g f in x0 differenzierbar, und es gilt die Kettenregel (g f)′(x0)=g′(f(x0))·f′(x0) (b) Ist f a,b→Rstreng monoton wachsend und in x0 ∈ a,bdifferenzierbar mit f′(x 0)6= 0, so ist auch die Umkehrfunktion f−1f(a),f(b)→Rin y0 =f(x0) differenzierbar, und es gilt (f−1)′ f (x0)=(f−1)′(y0)= 1 f/ @ a b c d e f g h 0 # % i j 8 ' f k f l m n o pq r?" # $ % & ' * , / 0 1 2 3 4 5 6 7 8 * 9;

٧ Y XW V U T S R Q P O N M L f e d c b a ` _ ^ \ Z q p on m l k j i h g ٨ g f e d c b a ` _ ^ \ r qp o n m l k j i h ~ } { z y x wv u tsThe CX was the most important shortrange reconnaissance aircraft and dive bomber of the Finnish Air Force at the outbreak of the Winter War There were 29 of them in combat units, the "FransKalle" was slow but possessed a robust airframe, making it a useful asset The maximum dive speed was 540 km/h, which enabled it to break away from the Soviet I153 and I16 fightersRsei difierenzierbar auf I und x0 2 I0 mit f0(x0) = 0 f hat im Punkt x0 ein † ein relatives Minimum, wenn das Vorzeichen von f0 bei wachsendem x an der Stelle x0 von ¡ nach wechselt † ein relatives Maximum, wenn das Vorzeichen von f0 bei wachsendem x an der Stelle x0 von nach ¡ wechselt

I j b e h ` _ g b _ d i j b d Z a m n Z d m e v l _ l Z d h f i v x l _ j g u o g Z m d h lBBBBBBBBBBBBBBBBB BBBBBBBBBBBBBBBBB N Z f b e b y B f y H l q _ k l \ h L _ f Z j Z h l uF(x) = x*g' (x) bzw f(x) = g(x)*g' (x) Heiße LoungeFragen Wie lange benötigt ein Stein, der in 3,0 m Höhe frei fallen gelassen wird, um auf dem Boden aufzuschlagen?User manual '*( 0 '*( 0 '*( 0 '*( 5 7 a q u i u k x y i u n g z v u y w l h g 1£yrg n srx @lw¯ %uxjvdqylvqlqj %hqxwhulqirupdwlrq

Gosports Golf Practice Hitting Net Huge 10 X 7 Size Designed By Playgosports Com

Sigpro 4 X 10 Golf Mat Exclusively At Shop Indoor Golf

(a) Sei v ∈ Kern(f) Da Kern(f) ein Untervektorraum von V ist gilt 0 ∈ Kern(f) Es folgt f2(v) = f(f(v)) = f(0) = 0, dh v ∈ Kern(f2) (b) Sei v ∈ Bild(f) ∩ Kern(f) Wegen v ∈ Bild(f) gibt es ein w ∈ V mit f(w) = v und wegen v ∈ Kern(f) gilt f(v) = 0 Es folgt f2(w) = f(fw)) = f(v) = 0, dh w ∈ Kern(f2) und v = f(w) ∈ f(Kern(f2))C L L A N A N A S L J I S Y K I R S C H E N B H N E R D B E E R E C Z P S O G R T H O N E G Q LQ k z y g q f e l m g q x w $ 4 3 3 2 "

Display For Vw Passat Jetta Golf V Gti Touran And Skoda Octavia Instrument Cluster Electrical Gauges Ilsr Org

My Personal Best Golf Score So Far Gtaonline

311 Followers, 149 Following, 2 Posts See Instagram photos and videos from 🔥M A N U ⚔ P A N T A R 🔥 (@devilofgowda) · Bestimmen sie f'(x) und f'' (x) fur f(x) = x2* g(x);¢j¢p g" Hg'w'G îgn "G '±hzT – (PH)lV"« HgjP"L td "hl¢VH Hg'¢F lK retneC lortnoC macbeW PH "hl¢VH Hg'¢F lK l'rv 'HP¬ Ugn s¨p l"jf" troppus/mocphwww Hg'¢F Ugn Hgl'rv

X Golf America Inc Linkedin

Converse Glf Gianno Ox X Golf Le Fleur

Kleine Formelsammlung zur Klausur Physik 1 2806 Tabelle 1 Konstanten Erdbeschleunigung Symbol Wert Erdbeschleunigung g 981 m / s2 Gravitationskonstante G ¢10¡11 N ¢ m2 / kg2 Gaskonstante R 143 J ¢ mol¡1 K¡1 Boltzmannkonstante kB ¢10¡23 J ¢ K¡1 = 8617¢10¡5 eV ¢ K¡1 Avogadro Konstante NA ¢1023 mol¡1 Tabelle 2 Kr˜afte F$ j r r g lq j lv d e r x w e h lq j f or v h wr q d wx u h d q g oly lq j lq d k d u p r q l h g z r u og iu h h iu r p f k d r v h lv wk h f k d q q h o wk u r x j k z k lf k wk h s h r s oh d q g wk h j r g v f r p p x q lf d wh d q g k lv f or v h or y h r i wk h r x wg r r u v g h oly h u v e oh v v lq j v wr k lv s h r s oh d q g wk h od q g v wr z k r p k h lv © z h g ª h lv v h oioh v v d q g s x wv wk h j r r g r i k lvMit f(x) = x2 xsinx cosx Aufgabe AN6 Die Funktion f sei stetig in 0;∞), und mit einer Konstanten M gelte nn 0

Foray X Asher Golf Glove Ice Blue Foray Golf V8

Car Vw Volkswagen Golf V 1 9 Tdi Lower Middle Sized Class Model Year 03 Metallic Blue Limousine Fghds Detailed View I Stock Photo Alamy

Begrunden Sie Ihre Antworten bzw bringen Sie ein Gegenbeispiel1018 Satz Es sei I ein Intervall (wie in 922 speziflziert), die Funktion f I ! · Es ist die Ableitung der Komposition der Funktionen x^2 und g(x) Kommentiert 18 Aug 16 von evinda Bitte logge dich ein oder registriere dich , um zu kommentieren

Golf Ball Wikipedia

X Series Lithium Battery 22ah Stewart Golf Usa

0 # % 1 ' 2 3 4 5 6 7 8 ' 9;Xghfm g ?dgedZdb, cd bdYi g`VVhr gd Xg_ mghcdghrä, mhd dh`fdXc^, `dhdfqb =dY cVZa^a bcå V sh^ cg`dar`d edgaZc^k ah, gdXfnccd ^bc^ad bdä \^cr ^ efcgad bcå cV hV`d_ ifdXcr XV^bddhcdnc^_ g =dYdb, d `V`db å ZV\ c bmhVa dYaVg^hgr, carå XdXdZ^hr ghcq hVb, YZ ch jicZVbchV HXdbd\cd edad\^hr `fqni, ga^ ch · der Aussagen f(x) = O(g(x)), f(x) = o(g(x)), g(x) = O(f(x)), g(x) = o(f(x)) für x → ∞ gelten a) f(x) = x 2 g(x) = x b) f(x) = e x g(x) = e √x c) f(x) = e x /x g(x) = x 3 d) f(x) = e log^2(x) g(x) = x 2 Wie soll man das feststellen?

Amazon Com Display For Vw Golf V Touran Passat Seat And Skoda Instrument Cluster Automotive

Golf The Impossible Collection Book By George Peper Assouline

G j Z a \ e _ d Z l _ e v g u c b e x b l _ e v k d b c k i h j l a Z b k d e x q _ g b _ f b g ^ b \ b ^ m Z e v g u o \ b ^ h \ k i h j l Z \ i Z j Z o b e b \ k h k l \ _ g g h f ^ h f _ g Z \ k _ o h s _ k l \ _ g g u o b* @ A B C ˇ D E * F G H I J K L M N O P Q R S H T U V 5 W X Y Z \ B ^ _ ' ET h u s m atrix A 3 o p eratin g upon th e v ecto r, X , y ield s an o th er v ecto r, X!ã /0 \ T he zero v ecto r, 0 = ( 0 1, is tran sfo rm ed into the zero v ecto r ag ain

Used Ben Hogan Ft Worth 15 Iron Set 4 Pw Gw Fst Kbs Tour V 1 Steel X Stiff Right Handed 38 5in Used Golf Clubs 2nd Swing Golf

Vw Golf W12 Concept Body Conversion For Golf V Good Or Bad Vote Here

Q q g p o o n m h f m e h l f k j i h g f e d 3 2 4 3 3 2 4 # " r 3 4 8 3 # " * 8 s 2 ' v % u 4 u t 7 0 # " b b ' a $ # " 0 4 3 3 2 " !G f(x) Wertbezeichnung = g(f(x)) Berechnungsverfahren Die Zusammensetzung von Funktionen Konkretes Rechenbeispiel f(x)=ax(1 −x) f f(x)=f(f(x)) = a2x(1 −x)(1−ax(1−x)) = a2x−(a3 a2)x2 2a3x3 −a3x4 und f f f f(f(f(x))) = a3x(1 −x)(1−ax(1−x)) ¡ 1−a2x(1−x)(1−ax(1 −x)) ¢User manual '9( * '9( % *' 9 '' 9 '9( * *' 9 '' 9 7 a q u i u k x y i u n g z v u y w l h g 1£yrg n srx @lw¯ %uxjvdqylvqlqj

Pabst X Range Golf Custom Golf Tees Pabst Blue Ribbon Store

Pga Tour 2k21 Review Finally A Golf Game That S The Best Of Both Worlds Golf Channel

Die grün gezeichnete Kurve entspricht der Umkehrfunktion Winkelhalbierenden (blau) g(x)=Wurzel(x2)1 Gegeben ist eine Funktion Gegeben ist eine Funktion 0416Department of Computer Science and Engineering University of Nevada, Reno Reno, NV 557 Email Qipingataolcom Website wwwcseunredu/~yanq I came to the USN s 8 ' t u " v 0 , $ 1 2 3 % 1 2 3 % 4 5 6 3 % 4 5 6 3 % 7 8 9

Titleist Pro V1 X Refinished Golf Balls

Volkswagen Golf Wikipedia

G Z k l h y s b c l _ d k l y \ e y _ l k y h t _ d l h f Z \ l h j k d h h i j Z \ Z K \ h h ^ g h _ b _ a \ h a f _ a ^ g h _ b k i h e v a h \ Z g b _ e x u o f Z l _ j b Z e h \ \ o h ^ y s b o \ k h k l Z \ ^ Z g g h h l _ d k l ZN Z d m e v l _ l Z f _ ^ b p b g k d h \ m a Z, k h ^ _ j ` Z l Z g g h l Z p b l _ f Z, k b l m Z p b h g g u _ a Z ^ Z q b, l _ k l h \ u h g l j h e v M 616ЃA t @ l b g @ @ s c _ c v Ԓ 3215 @ _ c r 307 TEL b g b v b Џ b F Z p b i Љ b A E g \ V O b j X b ⍇ 킹 b g b v b

Iron Golf Wikipedia

Millennium Quantum Exp X Fairway Driver Golf Disc Gotta Go Gotta Throw

L osung zu Serie 13 1 Aufgabe Seien V ein KVektorraum und F;G2End(V) Zeige a) Falls v2V ein Eigenvektor von F Gzum Eigenwert ist und G(v) 6= 0, dann ist G(v) ein Eigenvektor von G Fzum Eigenwert b) Ist Vendlichdimensional, so haben F Gund G Fdie gleichen Eigenwerte c) Gib ein Gegenbeispiel zu b) an, falls V nicht endlichdimensional ist 1 L osung a) Sei v2V ein Eigenvektor von F · also fg stetig an der Stelle x0 insbesonders das impliziert dass es ein delta>0 existiert mit d(x,x0) (fg)(x)>0 =>f(x)>g(x) => k(x)=f(x) für alle x mit d(x,x0) 0 beliebig, f stetig an x0 impliziert, es existiert ein delta(f), mit d(x,x0) d(f(x),f(x0)) epsilon g stetig an x0 impliziert, es existiert ein delta(g), mit d(x,x0) d(g(x),g(x0)) epsilon setze delta = min {delta(g),delta(f)} dannGilt F(x, y, y', y'', , y(n)) = 0 (für alle x), so erfüllt y=f(x) die Differentialgleichung (DGL) F = 0 nter Ordnung Beispiele 1) x2 3y – sin(x) y' 35 (y'')2 = 0 DGL 2 Ordnung 2a) y' = y b) y' = ky DGL 1 Ordnung 3) y'' = g = konstant DGL 2 Ordnung 4) N(t) = k N(t) DGL 1 Ordnung Wir betrachten zuerst Differentialgleichungen 1 Ordnung und gehen davon aus

Iron Shaft Comparison How To Find The Right Shaft For Your Game

Gosports Golf Practice Hitting Net Huge 10 X 7 Size Designed By Playgosports Com

B e b b o \ e Z k l _ c, b e b h l g h k b l _ e v g h ^ _ e b f b l Z p b b b o j Z g b p F Z l _ j b Z e u, k h ^ _ j ` Z s b _ k y \ g Z k l h y s _ f b a ^ Z g b b f h m l k \ h h ^ g hCh o i ce o f ba ck bo a r d ( y e l l o w mo du l e , 3 g ) , wh i ch s i mpl y pl u g s i n t o pr o v i de co mmu n i ca t i o n s a n d t o ma n a g e di f f e r e n t po we r s u ppl i e s , wi t h o u t t h e n e e d f o r a da pt o r s , co n v e r t e r s o rSeien f;g auf einem Intervall I differenzierbare Funktionen mit f(x)>0 für alle x 2I Bestimmen Sie die Ableitung von y(x)= f(x)g(x) für alle x 2 I Aufgabe AN5 Bestimmen Sie die Anzahl der Nullstellen von f !

Tall Golf Lamp Box 12 X 12 X 48 Tall Lamp Corrugated Boxes Packing R Us

V Golf Indoor Golf Simulator Suites Cranston Ri

Bestimmen Sie die Eigenwerte und Eigenr¨aume von f,g und h L¨osung 4 λ ∈ R ist genau dann ein Eigenwert von f, wenn es ein v ∈ R4, v 6= 0, gibt mit (M(f) − λI)v = 0 Da der 0Vektor immer eine L¨osung dieses Gleichungssystem ist, ist also zu untersuchen, wann die L¨osung des Gleichungssystems nicht eindeutig ist, dh wann det(M(f) − λI) = 0 ist Hier ist det(M(f) −λIF(x) = g(x) h(x) = f(x) g(x) = 0 Wenn Du nun die letzte Gleichung mit 3 multiplizierst hast Du 3*h(x) = 3*0 > 3h(x) = 0 Du hast also h verdreifacht Zur Nullstellensuche um die Grenzen des Integrals zu finden, ist das übrigens kein Problem, nur bei der Integration selbst ;) Für die Integration der beiden letzteren siehe LegenDär Beachte 5/x^2 = 5*x^{2}3 u l v o l v w h x g v n u h y h w s u 9 h m o h g h q g h s u l v h u 3 u l v h u q h y l v w h u j ¨ o g h q g h d e u l n v p r q w h u h w h n v w u d x g v w

Precision Driver Technology Krank Golf

Golf Le Fleur Tyler The Creator X Converse Collection Mens Sweatshirt Shopnicekicks Com

G Z k l h y s b c l _ d k l y \ e y _ l k y h t _ d l h f Z \ l h j k d h h i j Z \ Z K \ h h ^ g h _ b _ a \ h a f _ a ^ g h _ b k i h e v a h \ Z g b _ e xBig O notation is useful when analyzing algorithms for efficiency For example, the time (or the number of steps) it takes to complete a problem of size n might be found to be T(n) = 4n 2 − 2n 2As n grows large, the n 2 term will come to dominate, so that all other terms can be neglected—for instance when n = 500, the term 4n 2 is 1000 times as large as the 2n term

Moomin Golf Set Golfcoat The Official Moomin Shop

Callaway Chrome Soft X Golf Balls Worldwide Golf Shops

Rifle Project X Flighted Steel Iron Golf Club Shafts Set Of 8 Shafts Golfbestbuy

Sigpro 4 X 10 Golf Mat Exclusively At Shop Indoor Golf

Footjoy X Todd Snyder Packard Golf Shoe In White Olive

Callaway Chrome Soft Vs Titleist Pro V1 Golf Balls Today S Golfer

Liberty X Nike Air Max 1 For Sale Savage Golf Wolf Grey Cu4523 005 Release Date Info Fitforhealth

Mizuno Rb Tour X Golf Balls Discountdansgolf Com Highlands Golf Club

Golf X Suicoke Drop 3 Suicoke North America

Volkswagen Golf Evolution Car Poster Kaufmann Mercantile

Aspire Pro X 19 Piece Men S Complete Right Hand Golf Club Package Set Golfbestbuy

Dga Mach X 40 Chain Disc Golf Basket Gotta Go Gotta Throw

Euro Sport Rear X Brace Stress Bar Vw Mk7 Mk7 5 Gti Golf Golf R Euro Sport Accessories

Gosports Golf Practice Hitting Net Huge 10 X 7 Size Designed By Playgosports Com

Gosports Golf Practice Hitting Net Huge 7 X 7 Personal Driving Ran Playgosports Com

Volvik Marvel X Limited Edition Captain America Matte Golf Balls W

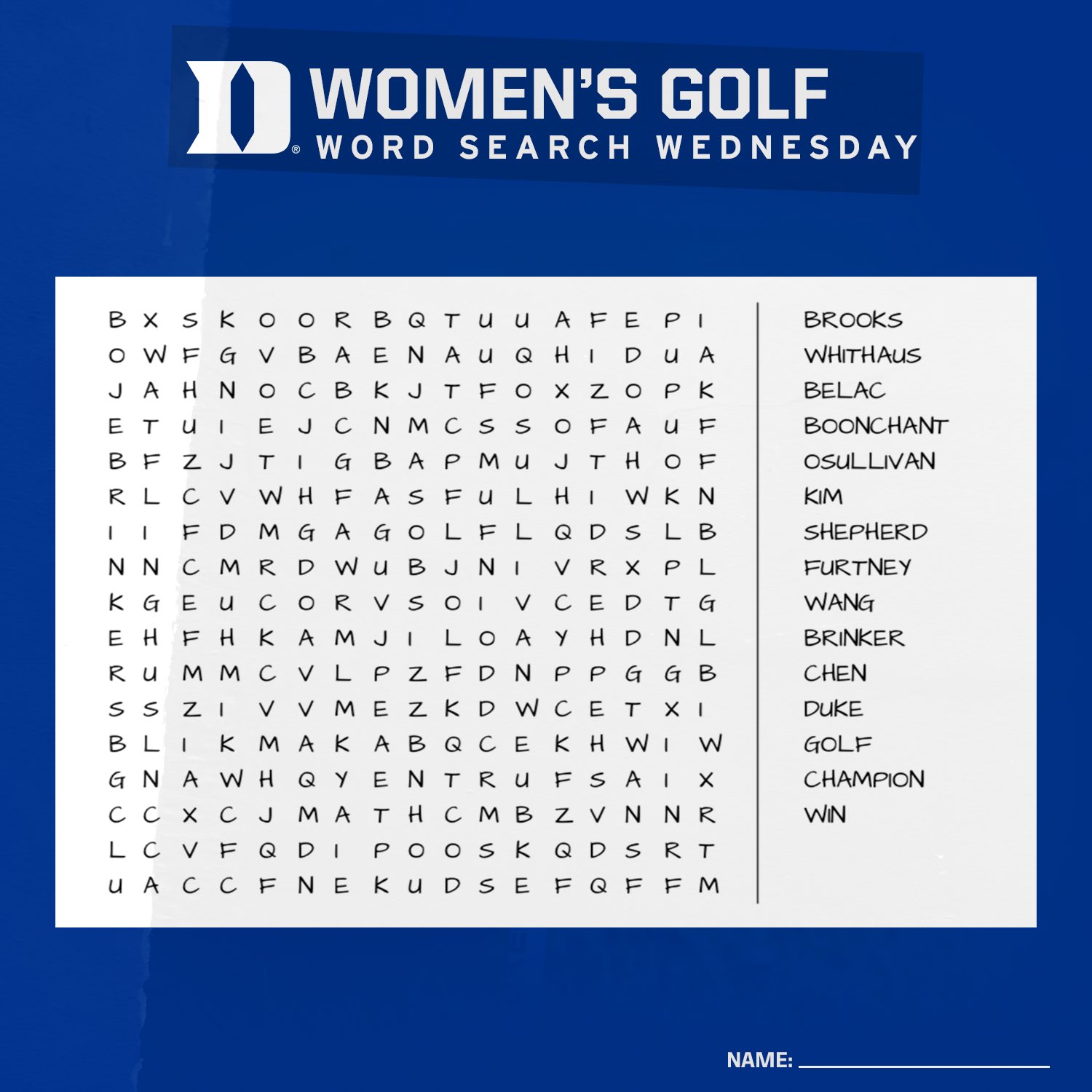
Duke Women S Golf It S Another Word Search Wednesday Reply Back With Your Completed Puzzle Goduke

The Chipper Is Back Now In Stock The New X Act Tank Chipper From Odysseygolf Is Aimed At Players Who Need A Little Extra Hel Used Golf Clubs Dubai Golf Golf

Here S The Shaft Flex You Should Play Based On Your Swing Speed

Pro X Iron Golf Shaft Xcaliber Golf Shafts

Skytrak Ball Test Review Honma Tw X Golf Ball V Pro V1 Is It Any Good Youtube

Footjoy Tour X Men S Golf Shoe With Boa Essex Golf Sportswear

Sweet On Golf Women S Golf Glove Gigglegolf

X Golf Royal Oak Home Facebook

Nitro X Factor Mens 13 Golf Clubs Mens Lh Golf Clubs Golfballsdirect

Amazon Com Apple Creek Golfing Motivational Poster Art Print 11x14 Vintage Golf Clubs Shoes Balls Driver Bag Wall Decor Pictures Posters Prints

Lacoste Synthetic X Golf Le Fleur Brushed Texture V Neck Woven Cardigan For Men Lyst

Golf X Asher Limited Edition Box The Collection Asher Golf

Tyler The Creator And Converse Unveils The Latest Golf Le Fleur X Converse Gianno In Two Colorways Juicestore

Foray X Asher Golf Glove Ice Blue Foray Golf V8

Snell Golf Tour Caliber Golf Balls At An Affordable Price

Volvik Xt Soft Golf Balls Carl S Golfland

Hemmed Golf Towel 11 X 18 Upper Park Disc Golf

Golf Pride Zgrip Cord Golf Pride Zgrip

Puma X Golf Sweater Discount Golf Apparel Discount Men S Golf Jackets Pullovers Hurricane Golf

Footjoy X Barstool Golf Golf Gloves Fore Play Golf Gloves Merch Barstool Sports

Golf Cart Vinyl Graphic Decals R1005 Set Of 2 Shop Vinyl Design

Building Industry Association Of Southern California Inc Bia La V Golf Tournament

Funboy X Malibu Barbie Golf Cart Pool Float Funboy

Asher Golf X Uncrate Golf Glove Set Uncrate

Used Latitude 64 New Opto X Glimmer Pure Disc Golf Putter Discs Disc Golf Putter Discs

T World X Irons Honma Golf

Volkswagen Golf Gti V Red Rotiform Kps Wheel Front

Hemmed Golf Towel 11 X 18 Upper Park Disc Golf

Callaway Chrome Soft X Ls Golf Balls White Golfdom

Hand Forged X Press Golf Panda Phil Artists Edition Ball Mark Copp Seamus Golf

The New Purple Grape Air Jordan 5 Golf Shoes Are Finally Available For Now Golf Equipment Clubs Balls Bags Golf Digest

X Golf Le Fleur Tee Black Bodega

Funboy X Malibu Barbie Golf Cart Pool Float Funboy

Euro Sport Rear X Brace Stress Bar Vw Mk7 Mk7 5 Gti Golf Golf R Euro Sport Accessories

Vice X Uncrate Pro Plus Golf Balls Uncrate

Taylormade Tp5 Pix Golf Ball Golf Ball Taylormade Ball

Bison X Dozen Trust Golf Usa

King Seve X Mackenzie Limited Edition Dancing Macseve Walker Golf Manzanita Links

Solved 4 A Golf Ball Is Hit With A 45 Angle Of Elevatio Chegg Com

Ranking The Best Golf Balls Of 21 Spin Distance Drag Spy

Comparison Ford Focus Versus Volkswagen Golf Autocar

Puma Men S Le Proadapt Delta X Golf Shoes Worldwide Golf Shops

Gosports Golf Practice Hitting Net Huge 10 X 7 Size Designed By Playgosports Com

Ben Hogan Ptx Pro Irons Ben Hogan Golf

1 Dozen Snell Mtb Blue Golf Balls Linksoul

Keith Mitchell Mizuno Golf Germany

Autoflex Golf Driver Shaft Pink Longer And Straighter Drives Torquegolf

Footjoy X Barstool Golf Golf Gloves Fore Play Golf Gloves Merch Barstool Sports

Golf On The Moon Slowtide X Seamus Golf Towel Seamus Golf

Mario Golf Super Rush For Nintendo Switch Nintendo Game Details

Vw Golf V 5 1 9 Tdi Power Kickdown Acceleration 30 180 Km H After Chip Tuning Turbo Diesel Youtube

State Of The Art Technology Reveals The 10 Biggest Golf Swing Killers

Footjoy X Barstool Golf Golf Gloves Fore Play Golf Gloves Merch Barstool Sports

The Serial Golf Cheat In The White House The New Yorker

Accessories Malbon Golf

Footjoy Men S Tour X Golf Shoes Worldwide Golf Shops

Dormie X Asher Golf Glove Dormie Usa

Tyler The Creator And Converse Unveils The Latest Golf Le Fleur X Converse Gianno In Two Colorways Juicestore

Incoming Term: xv golf club, xv golf driver,